“But Miss, I don’t even know which equation to use!”
Three top concerns of science teachers at the moment include:
- The GCSE required practicals
- The numeracy demand of the new GCSE
Last week I led a workshop with teachers from schools in Hertfordshire in an attempt to address these in tandem. Titled “teaching numeracy through physics practicals” we reflected on students’ difficulties with using maths in science and looked at some experiments which can help overcome these.
Starting with a lively discussion about “what do students find difficult about maths?” many commented on the increased level of mathematical demand posed by the new “Goved-up” specifications. Graphs and equations were felt to be particular issues, so here are my thoughts on how we can support students with these in particular.
1. Graphs – why do students suffer from graph-phobia when us science teachers love the instant visual hit of information that graphs give us?
Graphs as stories

Can we teach students to love graphs too by introducing them to some fun graphs?
A few examples like this one can begin to break down the antipathy some students have towards graphs. Thanks to www.truthfacts.com for these and many more!
Sketching their own graph stories could be the next step; no scale, just simply-labelled axes and a line, perhaps on a mini-whiteboard to develop confidence.
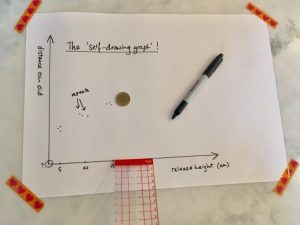
The self-drawing graph
For many students, constructing and using a scale is a real challenge and gets in the way of the importance of plotting a graph and seeing a relationship. To address this, the “self-drawing graph” can teach important lessons about variables, axes, uncertainty and anomalies – all without graph paper or the faff of a tricky scale! (I can’t take any credit for this – most of my ideas are borrowed!)
Axes – which way round?
The Hooke’s Law experiment (stretching springs) makes us think about the right way round to plot a graph of force against extension. Should the students plot force on the horizontal axis because that’s the independent variable in the experiment, or on the vertical axis so that the gradient represents a useful physical concept: stiffness? Similar arguments apply to the Ohm’s Law practical (investigating resistance).
These are not easy problems for science teachers to ponder, let alone Year 10 or 11 students, but make for good discussions in departments, especially perhaps in collaboration with the maths department.
2. Equations– learning, recalling, selecting, rearranging, and using them. Why do students find it so hard to apply what they know so well from maths lessons as soon as they walk into a science lab?
Our teachers tackled the ‘how far up a mountain could a chocolate bar’s-worth of energy propel you?’ challenge to highlight some of the mathematical terrors students can face when applying maths in science lessons.
Oomph and Grip
If you have come across the rope loop for demonstrating the effect on current of changing the voltage or resistance in a circuit, you’ll know how effective it can be for teaching students to get a real physical feel for the relationship I = V/R. (Note: perhaps a more conceptually helpful way round than V = IR). My colleague Colin describes this as “oomph and grip”. Simply put, most equations have a term which gives oomph or drives some effect (in this case, voltage or potential difference driving current) and grip which acts against the oomph (resistance here, working against the current).
Play around with easy numbers
We also explored the idea of using easy numbers to help students understand a relationship before ever seeing an equation. In fact, it’s possible to get to a position where the students can work out the equation for themselves – even with something relatively complicated such as v = u + at. We need to reinforce the relationship using simple numbers which can be handled with straightforward mental arithmetic.
It might go a bit like this:
A skateboarder accelerates at 3 m/s2. This means that every second she goes 3 m/s faster. So, from rest after one second she will be going at 3 m/s. After 2 seconds, 6 m/s. It will take her 10 seconds to reach 30 m/s. If she had already been going at 7 m/s to start with, she’ll have a speed of 10 m/s, 13 m/s, 16 m/s after 1, 2, 3 seconds….etc. Decelerating at 5 m/s2 her speed will decrease by 5 m/s every second. Ah, that’s why deceleration is also called negative acceleration and is a vector!
And so on…
We don’t need to bamboozle students with complex maths, difficult rearranging and unnecessarily tricky numbers. (And, by the way, let’s not feed them the fast food diet of ‘triangles’ either!) If we can present a numerical relationship in a way that connects it with a physical phenomenon (kinaesthetically, we might call it) and then reinforce that relationship with real (easy) numbers and a visual graphical appreciation I think we can give our students a deeper – and therefore more long-lasting – understanding.
Teachers are spending many evenings running ‘intervention’ or revision classes with year 11. A cynic might say they are simply teaching the whole course all over again because the students didn’t get it first time round. I say we should invest more time helping their understanding the first time round – moving from surface to deep learning as Shaun Allison (1) would put it – and use teachers’ after-school time running exciting science clubs instead…
This is not meant to be an exhaustive or prescriptive solution to the problem of students’ issues with maths in GCSE science, just a few practical ideas to help in the right direction. If you give any of these ideas a go, let me know how you get on! Or get in touch with me via www.astinconsulting.com if you want some more details.
Christina Astin, Physics Partners Trustee, Astin Consulting
This article first appeared in October 2018 on Christina’s website: www.astinconsulting.com/numeracy-challenges-in-gcse-science
Allison, Shaun (2017). Making Every Science Lesson Count: Six Principles to Support Great Science Teaching (Crown House Publishing)
